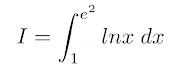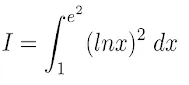Exercises for logarithmic and exponential functions involve tasks like graphing, solving equations, and applying these functions to real-world problems. These exercises help build a solid understanding of how logarithmic and exponential functions work and how they are used in various contexts.
The exercise
1- We consider the function g defined on the interval ]0, +∞[ as follows: g(x)= -xlnx + e
a - Analyze the variations of the function g and create a table of its variations.
b- Calculate g(e), then deduce the sign of g(x) over the interval ]0, +∞[.
2- We define the function f on the interval ]0, +∞[ as follows: f(x)= 1/2 (xlnx)² + ex — e and (Cf) is the graph of the function f in the coordinate plane associated with the orthogonal and homogeneous vectors (o; i乛,j乛) in which: ||乛i ||=2cm and ||乛j ||=4cm
a- Calculate the limit of f at 𝑫f and then interpret the result graphically.
b- Show that for any real number ]0, +∞[: f'(x)=(1/x)
c- Demonstrate that g is strictly decreasing on ]0, 1/e[ And strictly increasing on ]1/e, +∞ [ Then create a table of its variations.
3-
a- Calculate f(1), then show that f(x) = 0 has a unique solution in the interval ]0.11, 0.12 [.
b- Show that y=e(x-1) is the equation of the tangent line (T) to the curve (Cf) at the point with the abscissa 1.
c- Analyze the position of (T) with respect to (Cf).
d- Create (T) and (Cf).
4- We consider the function h defined by ]0,+∞[ by h(x)=| f(x)|, (Ch) graphically represent them in the previous coordinate system.
a- Explain how to construct (Ch) from the curve (Cf) Then create (Ch)
5-
|| ||